Average question in hindi, average tricks, average formula:-
We are jobriya.co.in is one of the largest study point where you can get complete details about jobs notification. Here we provide quantitative aptitude in Hindi. Now we provide average Question and answer in Hind. we provide average aptitude in Hindi, average short cuts,average online text in Hindi,average quiz test,Partnership formula,average problem with solutions in Hindi . here we provide all types of average question and answer in Hindi,average tricks,average short cuts,average formula, average question and answer pdf. here we also provide quantitative aptitude practices set. each set contain 30 question and total time will be 30 minutes. registred and without registred user can take online. quiz. if you have and complain related to any question please leave your feedback in comment box our support team resolve your problem as soon as possible.
Average Short Tricks, Average formula:-
= आंकडों का योगफल
या कुल राशि = औसत x आँकडों की संख्या
Average Short Tricks Type 1:-
= (n+1)(2n+1)
(जहाँ “n” अंतिम संख्या है)
d. प्रथम प्राकृतिक संख्याओं के घनों का औसत
= n(n+1)2
(जहाँ “n” अंतिम संख्या है)
Average Short Tricks Type 2:-
1. किसी कक्षा के 30 छात्रों की औसत आयु 14 वर्ष है, यदि एक अध्यापक की भी आयु शामिल कर ली जाये तो औसत आयु 15 वर्ष हो जाती है अध्यापक की आयु ज्ञात कीजिये
इसके लिये एक सामान्य सा सूत्र है और आप इसे बिना सूत्र के मौखिक भी निकाल सकते हैं वो बाद में जानेंगे पहले सूत्र
= नया औसत + सदस्यों की पुरानी संख्या x औसत में वृध्दि
= 15 + 30 x 1
= 45
2. चार व्यक्तियों का औसत वज़न 3 किलोग्राम बढ जाता है यदि 120 किलोग्राम वज़न वाले व्यक्ति के स्थान पर किसी और व्यक्ति को शामिल कर लिया जाता है
ये प्रश्न भी पहले वाले सूत्र से किया जा सकता है
= 120 + 4 x 3
= 132 किलोग्राम
3 . यदि कोई व्यक्ति किसी निश्चित दूरी को X कि0 मी0/ घंटा की रफ्तार से तथा उसी दूरी को Y किलोमीटर/घंटा की रफ्तार से तय करे तो उसकी औसत चाल क्या होगी ?
इसका सरलतम सूत्र है
= 2xy
और यदि वह तीन विभिन्न चालों से चले(xyz)
तो सूत्र होगा
= 3 xyz
xy+yz+zx
4. तीन लडकों की औसत आयु 15 वर्ष है यदि उनकी आयु 3:5:7 के अनुपात में है, सबसे छोटे लडके की आयु क्या होगी ? (SSC CGL 2014)
हल:
तीनों लडकों की कुल आयु होगी = 15 x 3 = 45 वर्ष
अब 45 वर्ष को 3 :5 : 7 के अनुपात में विभाजित कर लीजिये आपका उत्तर आ जायेगा
= 45
3+5+7
= 45
15
= 3
अब क्युंकि सबसे छोटे लडके की आयु पूछी गयी है इसलिये इसे सबसे छोटे वाले अनुपात से गुणा करेंगे
= 3 x 3 = 9 वर्ष
5. एक कक्षा के 40 छात्रों द्वारा प्राप्त अंको का औसत 86 है यदि 5 सर्वाधिक अंको को निकाल दिया जाये तो औसत एक अंक कम हो जाता है शीर्ष 5 छात्रों के औसत अंक बताइये (SSC CGL 2014)
हल:
सबसे पहले हम अभी अंको का योग निकालेंगे
= 86 x 40 = 3440
अब जो योग उन पाँच अंको को निकालने के बाद बनेगा वह है
= 35 x 85 = 2975
दोनों का अंतर = 3440 - 2975 = 465
ये है उन पाँच अंको का योग, अब इसका औसत निकालेंगे
= 465
5
= 93 उत्तर
6. चार बहनों की औसत आयु 7 वर्ष है यदि माँ की आयु शामिल कर दी जाये तो औसत आयु 6 वर्ष बढ जाती है तो माँ की आयु होगी (SSC CGL 2014)
हल:
सबसे पहले 4 बहनों की कुल आयु = 7 x 4 = 28
अब जब माँ की आयु शामिल कर ली जाती है तो औसत हो जाता है= 13
तथा कुल लोग = 4 बहन + माँ = 5
इसलिये कुल आयु = 13 x 5 = 65
अत: माँ की आयु = 65- 28 = 37 वर्ष
Short Trick से -
= नया औसत + सदस्यों की पुरानी संख्या x औसत में वृध्दि
= 13 + 4 x 6
= 37 वर्ष
7. किक्रेट के एक खिलाडी का 10 पारियों का कुछ औसत था 11 वीं पारी में उसने 108 रन बनाये तथा इससे उसकी औसत रन संख्या में 6 की बृध्दि हो गई अब उनकी औसत रन संख्या कितनी है
हल-
n वी पारी = 11
बनाये रन= 108
औसत में बृध्दि= 6
अभीष्ट औसत रन संख्या=आखिरी पारी n में बनाये रन -(n-1) x औसत में बृध्दि
=108 - (11-1) x 6
=108-60
= 48 रन
8. एक किक्रेट मैच में 6 खिलाडीयों की औसत रन संख्या 36 थी यदि इनमें से एक खिलाडी ने 16 रन बनाये हो, तो शेष खिलाडीयों की औसत रन संख्या कितना है
हल:
कुल रन = 36 x 6 = 216
इनमें से एक खिलाडी ने 16 रन बनाये हैं उन्हें घटा देते हैं
= 216- 16
= 200
अत: शेष खिलाडियों का औसत
= 200/5 = 40 उत्तर
इसके लिये एक सामान्य सा सूत्र है और आप इसे बिना सूत्र के मौखिक भी निकाल सकते हैं वो बाद में जानेंगे पहले सूत्र
= नया औसत + सदस्यों की पुरानी संख्या x औसत में वृध्दि
= 15 + 30 x 1
= 45
10. चार व्यक्तियों का औसत वज़न 3 किलोग्राम बढ जाता है यदि 120 किलोग्राम वज़न वाले व्यक्ति के स्थान पर किसी और व्यक्ति को शामिल कर लिया जाता है
ये प्रश्न भी पहले वाले सूत्र से किया जा सकता है
= 120 + 4 x 3
= 132 किलोग्राम
11 . यदि कोई व्यक्ति किसी निश्चित दूरी को X कि0 मी0/ घंटा की रफ्तार से तथा उसी दूरी को Y किलोमीटर/घंटा की रफ्तार से तय करे तो उसकी औसत चाल क्या होगी ?
इसका सरलतम सूत्र है
= 2xy
और यदि वह तीन विभिन्न चालों से चले(xyz)
तो सूत्र होगा
= 3 xyz
xy+yz+zx
12. तीन लडकों की औसत आयु 15 वर्ष है यदि उनकी आयु 3:5:7 के अनुपात में है, सबसे छोटे लडके की आयु क्या होगी ? (SSC CGL 2014)
हल:
तीनों लडकों की कुल आयु होगी = 15 x 3 = 45 वर्ष
अब 45 वर्ष को 3 :5 : 7 के अनुपात में विभाजित कर लीजिये आपका उत्तर आ जायेगा
= 45
3+5+7
= 45
15
= 3
अब क्युंकि सबसे छोटे लडके की आयु पूछी गयी है इसलिये इसे सबसे छोटे वाले अनुपात से गुणा करेंगे
= 3 x 3 = 9 वर्ष
13. एक कक्षा के 40 छात्रों द्वारा प्राप्त अंको का औसत 86 है यदि 5 सर्वाधिक अंको को निकाल दिया जाये तो औसत एक अंक कम हो जाता है शीर्ष 5 छात्रों के औसत अंक बताइये (SSC CGL 2014)
हल:
सबसे पहले हम अभी अंको का योग निकालेंगे
= 86 x 40 = 3440
अब जो योग उन पाँच अंको को निकालने के बाद बनेगा वह है
= 35 x 85 = 2975
दोनों का अंतर = 3440 - 2975 = 465
ये है उन पाँच अंको का योग, अब इसका औसत निकालेंगे
= 465
5
= 93 उत्तर
14. चार बहनों की औसत आयु 7 वर्ष है यदि माँ की आयु शामिल कर दी जाये तो औसत आयु 6 वर्ष बढ जाती है तो माँ की आयु होगी (SSC CGL 2014)
हल:
सबसे पहले 4 बहनों की कुल आयु = 7 x 4 = 28
अब जब माँ की आयु शामिल कर ली जाती है तो औसत हो जाता है= 13
तथा कुल लोग = 4 बहन + माँ = 5
इसलिये कुल आयु = 13 x 5 = 65
अत: माँ की आयु = 65- 28 = 37 वर्ष
Short Trick से -
= नया औसत + सदस्यों की पुरानी संख्या x औसत में वृध्दि
= 13 + 4 x 6
= 37 वर्ष
15. किक्रेट के एक खिलाडी का 10 पारियों का कुछ औसत था 11 वीं पारी में उसने 108 रन बनाये तथा इससे उसकी औसत रन संख्या में 6 की बृध्दि हो गई अब उनकी औसत रन संख्या कितनी है
हल-
n वी पारी = 11
बनाये रन= 108
औसत में बृध्दि= 6
अभीष्ट औसत रन संख्या=आखिरी पारी n में बनाये रन -(n-1) x औसत में बृध्दि
=108 - (11-1) x 6
=108-60
= 48 रन
16. एक किक्रेट मैच में 6 खिलाडीयों की औसत रन संख्या 36 थी यदि इनमें से एक खिलाडी ने 16 रन बनाये हो, तो शेष खिलाडीयों की औसत रन संख्या कितना है
हल:
कुल रन = 36 x 6 = 216
इनमें से एक खिलाडी ने 16 रन बनाये हैं उन्हें घटा देते हैं
= 216- 16
= 200
अत: शेष खिलाडियों का औसत
= 200/5 = 40 उत्तर
Average Online Quiz:-
Quiz-summary
0 of 31 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
Information
Check your Strength In Online Quantitative Aptitude
- Quiz Contain 30 Question
- Each Question Contain 1 marks and total time will be 20 Minutes.
- All Question Select on the basis of SSC,BANK, LIC exam pattern
- Registered User can check there rating among all candidate
- If you want to Register Click here
- User can manage profile,update there images.
- User can help other candidates
- In cause of any Problem, Please leave query on Comment Box
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Results
0 of 31 questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 points, (0)
| Average score |
|
| Your score |
|
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- 31
- Answered
- Review
-
Question 1 of 31
1. Question
One side of rectangular field is 15 meter and one of its diagonals is 17 meter. Then find the area of the field.
Correct
Let original length = x metres and original breadth = y metres.
Original area =xy m2New Length =120100x=65xNew Breadth =120100y=65y=>New Area =65x∗65y=>New Area =3625xyArea Difference=3625xy−xy=1125xyIncrease%=DiffernceActual∗100=11xy25∗1xy∗100=44%Original area =xy m2New Length =120100x=65xNew Breadth =120100y=65y=>New Area =65x∗65y=>New Area =3625xyArea Difference=3625xy−xy=1125xyIncrease%=DiffernceActual∗100=11xy25∗1xy∗100=44%
Incorrect
Let original length = x metres and original breadth = y metres.
Original area =xy m2New Length =120100x=65xNew Breadth =120100y=65y=>New Area =65x∗65y=>New Area =3625xyArea Difference=3625xy−xy=1125xyIncrease%=DiffernceActual∗100=11xy25∗1xy∗100=44%Original area =xy m2New Length =120100x=65xNew Breadth =120100y=65y=>New Area =65x∗65y=>New Area =3625xyArea Difference=3625xy−xy=1125xyIncrease%=DiffernceActual∗100=11xy25∗1xy∗100=44%
-
Question 2 of 31
2. Question
The area of a rectangle is 460 square metres. If the length is 15% more than the breadth, what is the breadth of the rectangular field ?
Correct
Explanation:
Let breadth =x metres.
Then length =(115x/100)metres.=x∗115×100=460×2=(460×100/115)x2=400x=20=x∗115×100=460×2=(460×100/115)x2=400x=20
Incorrect
Explanation:
Let breadth =x metres.
Then length =(115x/100)metres.=x∗115×100=460×2=(460×100/115)x2=400x=20=x∗115×100=460×2=(460×100/115)x2=400x=20
-
Question 3 of 31
3. Question
A rectangular field is to be fenced on three sides leaving a side of 20 feet uncovered.If the area of the field is 680 sq.ft, how many feet of fencing will be required ?
Correct
Explanation:
We are given with length and area, so we can find the breadth.
as Length * Breadth = Area
=> 20 * Breadth = 680
=> Breadth = 34 feetArea to be fenced = 2B + L = 2*34 + 20
= 88 feetIncorrect
Explanation:
We are given with length and area, so we can find the breadth.
as Length * Breadth = Area
=> 20 * Breadth = 680
=> Breadth = 34 feetArea to be fenced = 2B + L = 2*34 + 20
= 88 feet -
Question 4 of 31
4. Question
The perimeters of two squares are 40 cm and 32 cm. Find the perimeter of a third square whose area is equal to the difference of the areas of the two squares .
Correct
Explanation:
We know perimeter of square = 4(side)
So Side of first square = 40/4 = 10 cm
Side of second square = 32/4 = 8 cmArea of third Square = 10*10 - 8*8
= 36 cmSo side of third square = 6 [because area of square = side*side]
Perimeter = 4*Side = 4*6 = 24 cmIncorrect
Explanation:
We know perimeter of square = 4(side)
So Side of first square = 40/4 = 10 cm
Side of second square = 32/4 = 8 cmArea of third Square = 10*10 - 8*8
= 36 cmSo side of third square = 6 [because area of square = side*side]
Perimeter = 4*Side = 4*6 = 24 cm -
Question 5 of 31
5. Question
The Diagonals of two squares are in the ratio of 2:5. find the ratio of their areas.
Correct
Explanation:
Let the diagonals of the squares be 2x and 5x.
Then ratio of their areas will beArea of square=12∗Diagonal212∗2×2:12∗5x24x2:25×2=4:25Area of square=12∗Diagonal212∗2×2:12∗5x24x2:25×2=4:25
Incorrect
Explanation:
We know perimeter of square = 4(side)
So Side of first square = 40/4 = 10 cm
Side of second square = 32/4 = 8 cmArea of third Square = 10*10 - 8*8
= 36 cmSo side of third square = 6 [because area of square = side*side]
Perimeter = 4*Side = 4*6 = 24 cm -
Question 6 of 31
6. Question
A farmer wishes to start a 100 sq. m. rectangular vegetable garden. Since he has only 30 meter barbed wire, he fences three sides of the garden letting his house compound wall act as the fourth side fencing. Then find the dimension of the garden.
Correct
From the question, 2b+l = 30
=> l = 30-2bArea=100m2=>l×b=100=>b(30−2b)=100b2−15b+50=0=>(b−10)(b−5)=0Area=100m2=>l×b=100=>b(30−2b)=100b2−15b+50=0=>(b−10)(b−5)=0
b = 10 or b = 5
when b = 10 then l = 10
when b = 5 then l = 20
Since the garden is rectangular so we will take value of breadth 5.
So its dimensions are 20 m * 5 mIncorrect
From the question, 2b+l = 30
=> l = 30-2bArea=100m2=>l×b=100=>b(30−2b)=100b2−15b+50=0=>(b−10)(b−5)=0Area=100m2=>l×b=100=>b(30−2b)=100b2−15b+50=0=>(b−10)(b−5)=0
b = 10 or b = 5
when b = 10 then l = 10
when b = 5 then l = 20
Since the garden is rectangular so we will take value of breadth 5.
So its dimensions are 20 m * 5 m -
Question 7 of 31
7. Question
A courtyard is 25 meter long and 16 meter board is to be paved with bricks of dimensions 20 cm by 10 cm. The total number of bricks required is
Correct
Explanation:
Number of bricks =Courtyard area1 brick area=(2500×160020×10)=20000Number of bricks =Courtyard area1 brick area=(2500×160020×10)=20000Incorrect
Explanation:
Number of bricks =Courtyard area1 brick area=(2500×160020×10)=20000Number of bricks =Courtyard area1 brick area=(2500×160020×10)=20000 -
Question 8 of 31
8. Question
The length of a rectangle is three times of its width. If the length of the diagonal is
810−−√810 , then find the perimeter of the rectangle.Correct
Explanation:
Let Breadth = x cm,
then, Length = 3x cmx2+(3x)2=(810−−√)2=>10×2=640=>x=8×2+(3x)2=(810)2=>10×2=640=>x=8
Incorrect
Explanation:
Let Breadth = x cm,
then, Length = 3x cmx2+(3x)2=(810−−√)2=>10×2=640=>x=8×2+(3x)2=(810)2=>10×2=640=>x=8
-
Question 9 of 31
9. Question
What will be the cost of gardening 1 meter boundary around a rectangular plot having perimeter of 340 meters at the rate of Rs. 10 per square meter ?
Correct
Explanation:
In this question, we are having perimeter.
We know Perimeter = 2(l+b), right
So,
2(l+b) = 340
As we have to make 1 meter boundary around this, so
Area of boundary = ((l+2)+(b+2)-lb)
= 2(l+b)+4 = 340+4 = 344So required cost will be = 344 * 10 = 3440
Incorrect
Explanation:
In this question, we are having perimeter.
We know Perimeter = 2(l+b), right
So,
2(l+b) = 340
As we have to make 1 meter boundary around this, so
Area of boundary = ((l+2)+(b+2)-lb)
= 2(l+b)+4 = 340+4 = 344So required cost will be = 344 * 10 = 3440
-
Question 10 of 31
10. Question
A towel, when bleached, was found to have lost 20% of its length and 10% of its breadth. The percentage of decrease in area is ?
Correct
Explanation:
Let original length = x
and original width = y
Decrease in area will be=xy−(80×100×90y100)=(xy−1825xy)=725xyDecrease = (7xy25xy×100)%=28%=xy−(80×100×90y100)=(xy−1825xy)=725xyDecrease = (7xy25xy×100)%=28%
Incorrect
Explanation:
Let original length = x
and original width = y
Decrease in area will be=xy−(80×100×90y100)=(xy−1825xy)=725xyDecrease = (7xy25xy×100)%=28%=xy−(80×100×90y100)=(xy−1825xy)=725xyDecrease = (7xy25xy×100)%=28%
-
Question 11 of 31
11. Question
The perimeters of 5 squares are 24 cm, 32 cm, 40 cm, 76 cm and 80 cm respectively. The perimeter of another square equal in area to the sum of the area of these square is:
Correct
Clearly first we need to find the areas of the given squares, for that we need its side.
Side of sqaure = Perimeter/4
So sides are,
(244),(324),(404),(764),(804)=6,8,10,19,20Area of new square will be =[62+82+102+192+202]=36+64+100+361+400=961cm2Side of new Sqaure =961−−−√=31cmRequired perimeter =(4×31)=124cm(244),(324),(404),(764),(804)=6,8,10,19,20Area of new square will be =[62+82+102+192+202]=36+64+100+361+400=961cm2Side of new Sqaure =961=31cmRequired perimeter =(4×31)=124cm
Incorrect
Clearly first we need to find the areas of the given squares, for that we need its side.
Side of sqaure = Perimeter/4
So sides are,
(244),(324),(404),(764),(804)=6,8,10,19,20Area of new square will be =[62+82+102+192+202]=36+64+100+361+400=961cm2Side of new Sqaure =961−−−√=31cmRequired perimeter =(4×31)=124cm(244),(324),(404),(764),(804)=6,8,10,19,20Area of new square will be =[62+82+102+192+202]=36+64+100+361+400=961cm2Side of new Sqaure =961=31cmRequired perimeter =(4×31)=124cm
-
Question 12 of 31
12. Question
50 square stone slabs of equal size were needed to cover a floor area of 72 sq.m. Find the length of each stone slab.
Correct
Explanation:
Area of each slab =7250m2=1.44m2Length of each slab =1.44−−−−√=1.2m=120cm7250m2=1.44m2Length of each slab =1.44=1.2m=120cm
Incorrect
Explanation:
Area of each slab =7250m2=1.44m2Length of each slab =1.44−−−−√=1.2m=120cm7250m2=1.44m2Length of each slab =1.44=1.2m=120cm
-
Question 13 of 31
13. Question
What are the least number of square tiles required to pave the floor of a room 15 m 17 cm long and 9 m 2 cm broad ?
Correct
In this type of questions, first we need to calculate the area of tiles. With we can get by obtaining the length of largest tile.
Length of largest tile can be obtained from HCF of length and breadth.
So lets solve this,Length of largest tile = HCF of (1517 cm and 902 cm)
= 41 cmRequired number of tiles =
Area of floorArea of tile=(1517×90241×41)=814Area of floorArea of tile=(1517×90241×41)=814
Incorrect
In this type of questions, first we need to calculate the area of tiles. With we can get by obtaining the length of largest tile.
Length of largest tile can be obtained from HCF of length and breadth.
So lets solve this,Length of largest tile = HCF of (1517 cm and 902 cm)
= 41 cmRequired number of tiles =
Area of floorArea of tile=(1517×90241×41)=814Area of floorArea of tile=(1517×90241×41)=814
-
Question 14 of 31
14. Question
The area of a square is 69696 cm square. What will be its diagonal ?
Correct
If area is given then we can easily find side of a square as,
Side=69696−−−−−√=264cmwe know diagonal =2√×side=2√×264=1.414×264=373.296cmSide=69696=264cmwe know diagonal =2×side=2×264=1.414×264=373.296cm
Incorrect
If area is given then we can easily find side of a square as,
Side=69696−−−−−√=264cmwe know diagonal =2√×side=2√×264=1.414×264=373.296cmSide=69696=264cmwe know diagonal =2×side=2×264=1.414×264=373.296cm
-
Question 15 of 31
15. Question
If the ratio of the areas of two squares is 225:256, then the ratio of their perimeters is :
Correct
Explanation:
a2b2=2252561516< =>4a4b=4∗154∗16=1516=15:16a2b2=2252561516< =>4a4b=4∗154∗16=1516=15:16Incorrect
Explanation:
a2b2=2252561516< =>4a4b=4∗154∗16=1516=15:16a2b2=2252561516< =>4a4b=4∗154∗16=1516=15:16 -
Question 16 of 31
16. Question
The difference of the areas of two squares drawn on two line segments in 32 sq. cm. Find the length of the greater line segment if one is longer than the other by 2 cm.
Correct
Explanation:
Let the lengths of the line segments be x and x+2 cm
then,(x+2)2−x2=32×2+4x+4−x2=324x=28x=7cm(x+2)2−x2=32×2+4x+4−x2=324x=28x=7cm
Incorrect
Explanation:
Let the lengths of the line segments be x and x+2 cm
then,(x+2)2−x2=32×2+4x+4−x2=324x=28x=7cm(x+2)2−x2=32×2+4x+4−x2=324x=28x=7cm
-
Question 17 of 31
17. Question
The base of a triangle is 15 cm and height is 12 cm. The height of another triangle of double the area having the base 20 cm is :
Correct
Explanation:
Area of triangle, A1 = 12∗base∗height=12∗15∗12=90cm2Area of second triangle =2∗A1=180cm212∗20∗height=180=>height=18cmArea of triangle, A1 = 12∗base∗height=12∗15∗12=90cm2Area of second triangle =2∗A1=180cm212∗20∗height=180=>height=18cmIncorrect
Explanation:
Area of triangle, A1 = 12∗base∗height=12∗15∗12=90cm2Area of second triangle =2∗A1=180cm212∗20∗height=180=>height=18cmArea of triangle, A1 = 12∗base∗height=12∗15∗12=90cm2Area of second triangle =2∗A1=180cm212∗20∗height=180=>height=18cm -
Question 18 of 31
18. Question
The sides of a triangle are in the ratio of
12:13:1412:13:14
If the perimeter is 52 cm, then find the length of the smallest side.Correct
Ratio of sides =12:13:14=6:4:3Perimeter=52cmSo sides are =(52∗613)cm,(52∗413)cm,(52∗313)cmRatio of sides =12:13:14=6:4:3Perimeter=52cmSo sides are =(52∗613)cm,(52∗413)cm,(52∗313)cm
a = 24 cm, b = 16 cm and c = 12 cm
Length of the smallest side = 12 cmIncorrect
Ratio of sides =12:13:14=6:4:3Perimeter=52cmSo sides are =(52∗613)cm,(52∗413)cm,(52∗313)cmRatio of sides =12:13:14=6:4:3Perimeter=52cmSo sides are =(52∗613)cm,(52∗413)cm,(52∗313)cm
a = 24 cm, b = 16 cm and c = 12 cm
Length of the smallest side = 12 cm -
Question 19 of 31
19. Question
The height of an equilateral triangle is 10 cm. find its area.
Correct
Explanation:
Let each side be a cm, then(a2)2+102=a2< =>(a2−a24)=100< =>3a24=100a2=4003Area=3√4∗a2=(3√4∗4003)cm2=1003√cm2(a2)2+102=a2< =>(a2−a24)=100< =>3a24=100a2=4003Area=34∗a2=(34∗4003)cm2=1003cm2
Incorrect
Explanation:
Let each side be a cm, then(a2)2+102=a2< =>(a2−a24)=100< =>3a24=100a2=4003Area=3√4∗a2=(3√4∗4003)cm2=1003√cm2(a2)2+102=a2< =>(a2−a24)=100< =>3a24=100a2=4003Area=34∗a2=(34∗4003)cm2=1003cm2
-
Question 20 of 31
20. Question
If the area of a square with the side a is equal to the area of a triangle with base a, then the altitude of the triangle is.
Correct
Explanation:
We know area of square =a2Area of triangle =12∗a∗h=>12∗a∗h=a2=>h=2aWe know area of square =a2Area of triangle =12∗a∗h=>12∗a∗h=a2=>h=2aIncorrect
Explanation:
We know area of square =a2Area of triangle =12∗a∗h=>12∗a∗h=a2=>h=2aWe know area of square =a2Area of triangle =12∗a∗h=>12∗a∗h=a2=>h=2a -
Question 21 of 31
21. Question
What will be the ratio between the area of a rectangle and the area of a triangle with one of the sides of the rectangle as base and a vertex on the opposite side of the rectangle ?
Correct
Explanation:
As far as questions of Area or Volume and Surface area are concerned, it is all about formulas and very little logic. So its a sincere advice to get all formulas remembered before solving these questions.Lets solve this,
Area of rectangle =l∗bArea of triangle =12l∗bRatio =l∗b:12l∗b=1:12=2:1Area of rectangle =l∗bArea of triangle =12l∗bRatio =l∗b:12l∗b=1:12=2:1
Incorrect
Explanation:
As far as questions of Area or Volume and Surface area are concerned, it is all about formulas and very little logic. So its a sincere advice to get all formulas remembered before solving these questions.Lets solve this,
Area of rectangle =l∗bArea of triangle =12l∗bRatio =l∗b:12l∗b=1:12=2:1Area of rectangle =l∗bArea of triangle =12l∗bRatio =l∗b:12l∗b=1:12=2:1
-
Question 22 of 31
22. Question
The area of rhombus is 150 cm square. The length of one of the its diagonals is 10 cm. The length of the other diagonal is:
Correct
Option D
Explanation:
We know the product of diagonals is 1/2*(product of diagonals)Let one diagonal be d1 and d2
So as per question12∗d1∗d2=15012∗10∗d2=150d2=1505=3012∗d1∗d2=15012∗10∗d2=150d2=1505=30
Incorrect
Option D
Explanation:
We know the product of diagonals is 1/2*(product of diagonals)Let one diagonal be d1 and d2
So as per question12∗d1∗d2=15012∗10∗d2=150d2=1505=3012∗d1∗d2=15012∗10∗d2=150d2=1505=30
-
Question 23 of 31
23. Question
A triangle and a parallelogram are constructed on the same base such that their areas are equal. If the altitude of the parallelogram is 100 m , then the altitude of the triangle is.
Correct
Let the triangle and parallelogram have common base b,
let the Altitude of triangle is h1 and of parallelogram is h2(which is equal to 100 m), thenArea of triangle =12∗b∗h1Area of rectangle =b∗h2As per question 12∗b∗h1=b∗h212∗b∗h1=b∗100h1=100∗2=200mArea of triangle =12∗b∗h1Area of rectangle =b∗h2As per question 12∗b∗h1=b∗h212∗b∗h1=b∗100h1=100∗2=200m
Incorrect
Let the triangle and parallelogram have common base b,
let the Altitude of triangle is h1 and of parallelogram is h2(which is equal to 100 m), thenArea of triangle =12∗b∗h1Area of rectangle =b∗h2As per question 12∗b∗h1=b∗h212∗b∗h1=b∗100h1=100∗2=200mArea of triangle =12∗b∗h1Area of rectangle =b∗h2As per question 12∗b∗h1=b∗h212∗b∗h1=b∗100h1=100∗2=200m
-
Question 24 of 31
24. Question
Find the circumference of a circle, whose area is 24.64 meter sqaure
Correct
Explanation:
Area of Square =π∗r2=>π∗r2=24.64=>r2=24.6422∗7=>r2=7.84=>r=7.84−−−−√=>r=2.8Circumference =2π∗r=2∗227∗2.8=17.60mArea of Square =π∗r2=>π∗r2=24.64=>r2=24.6422∗7=>r2=7.84=>r=7.84=>r=2.8Circumference =2π∗r=2∗227∗2.8=17.60mIncorrect
Explanation:
Area of Square =π∗r2=>π∗r2=24.64=>r2=24.6422∗7=>r2=7.84=>r=7.84−−−−√=>r=2.8Circumference =2π∗r=2∗227∗2.8=17.60mArea of Square =π∗r2=>π∗r2=24.64=>r2=24.6422∗7=>r2=7.84=>r=7.84=>r=2.8Circumference =2π∗r=2∗227∗2.8=17.60m -
Question 25 of 31
25. Question
The wheel of a motorcycle, 70 cm in diameter makes 40 revolutions in every 10 seconds. What is the speed of the motorcycle in km/hr
Correct
Explanation:
In this type of question, we will first calculate the distance covered in given time.
Distance covered will be, Number of revolutions * CircumferenceSo we will be having distance and time, from which we can calculate the speed. So let solve.
Radius of wheel = 70/2 = 35 cm
Distance covered in 40 revolutions will be40 * Circumference =40 * 2*\pi*r =40∗2∗227∗35=8800cm=8800100m=88mDistance covered in 1 sec =8810=8.8mSpeed=8.8m/s=8.8∗185=31.68km/hr40 * Circumference =40 * 2*\pi*r =40∗2∗227∗35=8800cm=8800100m=88mDistance covered in 1 sec =8810=8.8mSpeed=8.8m/s=8.8∗185=31.68km/hr
Incorrect
Explanation:
In this type of question, we will first calculate the distance covered in given time.
Distance covered will be, Number of revolutions * CircumferenceSo we will be having distance and time, from which we can calculate the speed. So let solve.
Radius of wheel = 70/2 = 35 cm
Distance covered in 40 revolutions will be40 * Circumference =40 * 2*\pi*r =40∗2∗227∗35=8800cm=8800100m=88mDistance covered in 1 sec =8810=8.8mSpeed=8.8m/s=8.8∗185=31.68km/hr40 * Circumference =40 * 2*\pi*r =40∗2∗227∗35=8800cm=8800100m=88mDistance covered in 1 sec =8810=8.8mSpeed=8.8m/s=8.8∗185=31.68km/hr
-
Question 26 of 31
26. Question
If the radius of a circle is diminished by 10%, then the area is diminished by
Correct
Let the original radius be R cm. New radius = 2R
Area=πR2New Area =π2R2=4πR2Increase in area =(4πR2−πR2)=3πR2Increase percent =3πR2πR2∗100=300%Area=πR2New Area =π2R2=4πR2Increase in area =(4πR2−πR2)=3πR2Increase percent =3πR2πR2∗100=300%
Incorrect
Let the original radius be R cm. New radius = 2R
Area=πR2New Area =π2R2=4πR2Increase in area =(4πR2−πR2)=3πR2Increase percent =3πR2πR2∗100=300%Area=πR2New Area =π2R2=4πR2Increase in area =(4πR2−πR2)=3πR2Increase percent =3πR2πR2∗100=300%
-
Question 27 of 31
27. Question
If the circumference of a circle increases from 4pi to 8 pi, what change occurs in the area ?
Correct
2πR1=4π=>R1=22πR2=8π=>R2=4Original Area =4π∗22=16πNew Area =4π∗42=64π2πR1=4π=>R1=22πR2=8π=>R2=4Original Area =4π∗22=16πNew Area =4π∗42=64π
Incorrect
2πR1=4π=>R1=22πR2=8π=>R2=4Original Area =4π∗22=16πNew Area =4π∗42=64π2πR1=4π=>R1=22πR2=8π=>R2=4Original Area =4π∗22=16πNew Area =4π∗42=64π
-
Question 28 of 31
28. Question
The ratio between the length and the breadth of a rectangular park is 3 : 2. If a man cycling along the boundary of the park at the speed of 12 km/hr completes one round in 8 minutes, then the area of the park (in sq. m) is:
Correct
Explanation:
Perimeter = Distance covered in 8 min. = 
12000 x 8 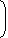 m = 1600 m.
m = 1600 m.60 Let length = 3x metres and breadth = 2x metres.
Then, 2(3x + 2x) = 1600 or x = 160.
 Length = 480 m and Breadth = 320 m.
Length = 480 m and Breadth = 320 m. Area = (480 x 320) m2 = 153600 m2.Incorrect
Area = (480 x 320) m2 = 153600 m2.Incorrect
Explanation:
Perimeter = Distance covered in 8 min. = 
12000 x 8 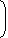 m = 1600 m.
m = 1600 m.60 Let length = 3x metres and breadth = 2x metres.
Then, 2(3x + 2x) = 1600 or x = 160.
 Length = 480 m and Breadth = 320 m.
Length = 480 m and Breadth = 320 m. Area = (480 x 320) m2 = 153600 m2.
Area = (480 x 320) m2 = 153600 m2. -
Question 29 of 31
29. Question
The ratio between the length and the breadth of a rectangular park is 3 : 2. If a man cycling along the boundary of the park at the speed of 12 km/hr completes one round in 8 minutes, then the area of the park (in sq. m) is: Correct
Explanation:
Perimeter = Distance covered in 8 min. = 
12000 x 8 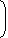 m = 1600 m.
m = 1600 m.60 Let length = 3x metres and breadth = 2x metres.
Then, 2(3x + 2x) = 1600 or x = 160.
 Length = 480 m and Breadth = 320 m.
Length = 480 m and Breadth = 320 m. Area = (480 x 320) m2 = 153600 m2.Incorrect
Area = (480 x 320) m2 = 153600 m2.Incorrect
Explanation:
Perimeter = Distance covered in 8 min. = 
12000 x 8 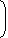 m = 1600 m.
m = 1600 m.60 Let length = 3x metres and breadth = 2x metres.
Then, 2(3x + 2x) = 1600 or x = 160.
 Length = 480 m and Breadth = 320 m.
Length = 480 m and Breadth = 320 m. Area = (480 x 320) m2 = 153600 m2.
Area = (480 x 320) m2 = 153600 m2. -
Question 30 of 31
30. Question
An error 2% in excess is made while measuring the side of a square. The percentage of error in the calculated area of the square is:
Correct
Explanation:
100 cm is read as 102 cm.
 A1 = (100 x 100) cm2 and A2 (102 x 102) cm2.
A1 = (100 x 100) cm2 and A2 (102 x 102) cm2.(A2 - A1) = [(102)2 - (100)2]
= (102 + 100) x (102 - 100)
= 404 cm2.
 Percentage error =
Percentage error =
404 x 100  %
%= 4.04% 100 x 100 Incorrect
Explanation:
100 cm is read as 102 cm.
 A1 = (100 x 100) cm2 and A2 (102 x 102) cm2.
A1 = (100 x 100) cm2 and A2 (102 x 102) cm2.(A2 - A1) = [(102)2 - (100)2]
= (102 + 100) x (102 - 100)
= 404 cm2.
 Percentage error =
Percentage error =
404 x 100  %
%= 4.04% 100 x 100 -
Question 31 of 31
31. Question
The percentage increase in the area of a rectangle, if each of its sides is increased by 20% is:
Correct
Explanation:
Let original length = x metres and original breadth = y metres.
Original area = (xy) m2.
New length = 
120 x  m
m= 
6 x  m.
m.100 5 New breadth = 
120 y  m
m= 
6 y  m.
m.100 5 New Area = 
6 x x 6 y  m2
m2= 
36 xy  m2.
m2.5 5 25 The difference between the original area = xy and new-area 36/25 xy is
= (36/25)xy - xy
= xy(36/25 - 1)
= xy(11/25) or (11/25)xy
 Increase % =
Increase % =
11 xy x 1 x 100 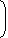 %
%= 44%. 25 xy Incorrect
Explanation:
Let original length = x metres and original breadth = y metres.
Original area = (xy) m2.
New length = 
120 x 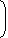 m
m= 
6 x 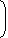 m.
m.100 5 New breadth = 
120 y 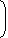 m
m= 
6 y  m.
m.100 5 New Area = 
6 x x 6 y  m2
m2= 
36 xy  m2.
m2.5 5 25 The difference between the original area = xy and new-area 36/25 xy is
= (36/25)xy - xy
= xy(36/25 - 1)
= xy(11/25) or (11/25)xy
 Increase % =
Increase % =
11 xy x 1 x 100  %
%= 44%. 25 xy
