Least Common Multiple Questions,Least Common Factor Questions:-
If you are looking online plate form where you can practice Quantitative aptitude question and answer for SSC Exam,Bank Exam, LIC AO, UP SI and other post. then you are at right place here i am going to share HCF, LCM question and answer in Hindi pdf . each question carry 1 marks and time will be for 30 question is 30 minutes. if you are looking for least common multiple questions,least common factor questions,lowest common multiple questions stop at jobriya.co.in
What Is HCF:- Highest Common Factor(HCF) of two or more numbers is the greatest number which divides each of them exactly.
- How to Calculate HCF:-
Step 1: Express each number as a product of prime factors.
Step 2: HCF is the product of all common prime factors using the least power of each common prime factor.
Example 1: Find out HCF of 60 and 75
Step 1 : Express each number as a product of prime factors.
60 = 22 × 3 × 5
75 = 3 × 52
Step 2: HCF is the product of all common prime factors using the least power of each common prime factor.
Here, common prime factors are 3 and 5
The least power of 3 here = 3
The least power of 5 here = 5
Hence, HCF = 3 × 5 = 15
Example 2: Find out HCF of 36, 24 and 12
Step 1: Express each number as a product of prime factors.
36 = 22 × 32
24 = 23 × 3
12 = 22 × 3
SO HCF is 23 × 3=12
- How to find out HCF - by dividing the numbers
Step 1: Write the given numbers in a horizontal line separated by commas.
Step 2: Divide the given numbers by the smallest prime number (write in the left side) which can exactly divide all the given numbers.
Step 3: Write the quotients in a line below the first.
Step 4: Repeat the process until we reach a stage where no common prime factor exists for all the numbers.
Step 5: We can see that the factors mentioned in the left side clearly divides all the numbers exactly and they are common prime factors. Their product is the HCF
Example 1: Find out HCF of 60 and 75
| 3 | 60,75 |
| 5 | 20,25 |
| 4,5 |
Hence HCF = 3 × 5 = 15.
- How to find out HCF using division method
To find out HCF of two given numbers using division method,
Step 1: Divide the larger number by the smaller number.
Step 2: Divisor of step 1 is divided by its remainder.
Step 3: Divisor of step 2 is divided by its remainder. Continue this process till we get zero as remainder.
Step 4: Divisor of the last step is the HCF.
To find out HCF of three given numbers using division method,
Step 1: Find out HCF of any two numbers.
Step 2: Find out the HCF of the third number and the HCF obtained in step 1.
Step 3: HCF obtained in step 2 will be the HCF of the three numbers.
In a similar way as explained for three numbers, we can find out HCF of more than three numbers also using division method.
Example 3: Find out HCF of 3556 and 3224

Hence HCF of 3556 and 3224 = 4
What Is Least Common Multiple (LCM):-
Least Common Multiple (LCM) of two or more numbers is the smallest number that is a multiple of all the numbers.
Example: LCM of 3 and 4 = 12 because 12 is the smallest number which is a multiple of both 3 and 4 (In other words, 12 is the smallest number which is divisible by both 3 and 4)
- How to find out LCM using prime factorization method
Step 1 : Express each number as a product of prime factors.
Step 2 : LCM = The product of highest powers of all prime factors.
Example 1: Find out LCM of 8 and 14
Step 1 : Express each number as a product of prime factors. (Reference: Prime Factorization)
8 = 23
14 = 2 × 7
Step 2 : LCM = The product of highest powers of all prime factors.
Here the prime factors are 2 and 7
The highest power of 2 here = 23
The highest power of 7 here = 7
Hence LCM = 23 × 7 = 56
Example : Find out LCM of 18, 24, 9, 36 and 90
Step 1 : Express each number as a product of prime factors.
18 = 2 × 32
24 = 23 × 3
9 = 32
36 = 23 × 32
90 = 2 × 5 × 32
Step 2 : LCM = The product of highest powers of all prime factors.
Here the prime factors are 2, 3 and 5
The highest power of 2 here = 23
The highest power of 3 here = 32
The highest power of 5 here = 5
Hence LCM = 23 × 32 × 5 = 360
How to find out LCM using division Method (shortcut method)
Step 1: Write the given numbers in a horizontal line separated by commas.
Step 2: Divide the given numbers by the smallest prime number which can exactly divide at least two of the given numbers.
Step 3: Write the quotients and undivided numbers in a line below the first.
Step 4: Repeat the process until we reach a stage where no prime factor is common to any two numbers in the row.
Step 5: LCM = The product of all the divisors and the numbers in the last line.
Example : Find out LCM of 8 and 14
| 2 | 8,14 |
| 4,7 |
Hence Least common multiple (L.C.M) of 8 and 14
= 2 × 4 × 7
= 56
Example : Find out LCM of 18, 24, 9, 36 and 90
| 2 | 18,24,9,36,90 |
| 2 | 9,12,9,18,45 |
| 3 | 9,6,9,9,45 |
| 3 | 3,2,3,3,15 |
| 1,2,1,1,5 |
Hence Least common multiple (L.C.M) of 18, 24, 9, 36 and 90
= 2 × 2 × 3 × 3 × 2 × 5
= 360
Ex. Find the H.C.F. and L.C.M. of 0.63, 1.05 and 2.1.
Sol. Making the same number of decimal places, the given numbers are 0.63, 1.05 and 2.10. Without decimal places, these numbers are 63, 105 and 210.
Now, H.C.F. of 63, 105 and 210 is 21.
H.C.F. of 0.63, 1.05 and 2.1 is 0.21.
L.C.M. of 63, 105 and 210 is 630.
L.C.M. of 0.63, 1.05 and 2.1 is 6.30.
Ex. 1. Find the H.C.F. of 23 X 32 X 5 X 74, 22 X 35 X 52 X 73,23 X 53 X 72
Sol. The prime numbers common to given numbers are 2,5 and 7.
H.C.F. = 22 x 5 x72 = 980.
Ex. 2. Find the H.C.F. of 108, 288 and 360.
Sol. 108 = 22 x 33, 288 = 25 x 32 and 360 = 23 x 5 x 32.
H.C.F. = 22 x 32 = 36.
Ex. Two numbers are in the ratio of 15:11. If their H.C.F. is 13, find the numbers.
Sol. Let the required numbers be 15.x and llx.
Then, their H.C.F. is x. So, x = 13.
The numbers are (15 x 13 and 11 x 13) i.e., 195 and 143.
Ex. 11. TheH.C.F. of two numbers is 11 and their L.C.M. is 693. If one of the
numbers is 77,find the other.
Sol. Other number = 11 X 693 = 99
77
Ex. Find the greatest possible length which can be used to measure exactly the lengths 4 m 95 cm, 9 m and 16 m 65 cm.
Sol. Required length = H.C.F. of 495 cm, 900 cm and 1665 cm.
495 = 32 x 5 x 11, 900 = 22 x 32 x 52, 1665 = 32 x 5 x 37.
H.C.F. = 32 x 5 = 45.
Hence, required length = 45 cm.
Ex. Find the largest number of four digits exactly divisible by 12,15,18 and 27.
Sol. The Largest number of four digits is 9999.
Required number must be divisible by L.C.M. of 12,15,18,27 i.e. 540.
On dividing 9999 by 540,we get 279 as remainder .
Required number = (9999-279) = 9720.
Ex.Find the smallest number of five digits exactly divisible by 16,24,36 and 54.
Sol. Smallest number of five digits is 10000.
Required number must be divisible by L.C.M. of 16,24,36,54 i.e 432,
On dividing 10000 by 432,we get 64 as remainder.
Required number = 10000 +( 432 – 64 ) = 10368.
Ex. Find the least number which when divided by 20,25,35 and 40 leaves remainders 14,19,29 and 34 respectively.
Sol. Here,(20-14) = 6,(25 – 19)=6,(35-29)=6 and (40-34)=6.
Required number = (L.C.M. of 20,25,35,40) – 6 =1394.
Ex.Find the least number which when divided by 5,6,7, and 8 leaves a remainder 3, but when divided by 9 leaves no remainder .
Sol. L.C.M. of 5,6,7,8 = 840.
Required number is of the form 840k + 3 Least value of k for which (840k + 3) is divisible by 9 is k = 2.
Required number = (840 X 2 + 3)=1683
Ex.The traffic lights at three different road crossings change after every 48 sec., 72 sec and 108 sec.respectively .If they all change simultaneously at 8:20:00 hours,then at what time they again change simultaneously .
Sol. Interval of change = (L.C.M of 48,72,108)sec.=432sec.
So, the lights will agin change simultaneously after every 432 seconds i.e,7
min.12sec
Hence , next simultaneous change will take place at 8:27:12 hrs.
LCM HCM Quiz:-
Quiz-summary
0 of 30 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
Information
Check your Strength In Online Quantitative Aptitude
- Quiz Contain 30 Question
- Each Question Contain 1 marks and total time will be 30 Minutes.
- All Question Select on the basis of SSC,BANK, LIC exam pattern
- Registered User can check there rating among all candidate
- User can manage profile,update there images.
- User can help other candidates
- In cause of any Problem, Please leave query on Comment Box.
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Results
0 of 30 questions answered correctly
Your time:
Time has elapsed
You have reached 0 of 0 points, (0)
| Average score |
|
| Your score |
|
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
- 26
- 27
- 28
- 29
- 30
- Answered
- Review
-
Question 1 of 30
1. Question
Find the HCF of
22×32×72,2×34×722×32×72,2×34×7
Correct
HCF is Highest common factor, so we need to get the common highest factors among given values. So we got
2 * 3*3 * 7Incorrect
HCF is Highest common factor, so we need to get the common highest factors among given values. So we got
2 * 3*3 * 7 -
Question 2 of 30
2. Question
Find the HCF of 54, 288, 360
Correct
Lets solve this question by factorization method.
18=2×32,288=25×32,360=23×32×518=2×32,288=25×32,360=23×32×5
So HCF will be minimum term present in all three, i.e.
2×32=18
Incorrect
Lets solve this question by factorization method.
18=2×32,288=25×32,360=23×32×518=2×32,288=25×32,360=23×32×5
So HCF will be minimum term present in all three, i.e.
2×32=18
-
Question 3 of 30
3. Question
Reduce 368575368575 to the lowest terms.
Correct
We can do it easily by in two steps
Step1: We get the HCF of 368 and 575 which is 23
Step2: Divide both by 23, we will get the answer 16/25Incorrect
We can do it easily by in two steps
Step1: We get the HCF of 368 and 575 which is 23
Step2: Divide both by 23, we will get the answer 16/25 -
Question 4 of 30
4. Question
Reduce 803876803876 to the lowest terms
Correct
HCF of 803 and 876 is 73, Divide both by 73, We get the answer 11/12
Incorrect
HCF of 803 and 876 is 73, Divide both by 73, We get the answer 11/12
-
Question 5 of 30
5. Question
HCF of 22×32×52,24×34×53×1122×32×52,24×34×53×11 is
Correct
As in HCF we will choose the minimum common factors among the given.. So answer will be third option
Incorrect
As in HCF we will choose the minimum common factors among the given.. So answer will be third option
-
Question 6 of 30
6. Question
What will be the LCM of 8, 24, 36 and 54
Correct
LCM of 8-24-36-54 will be
2*2*2*3*3*3 = 216Incorrect
LCM of 8-24-36-54 will be
2*2*2*3*3*3 = 216 -
Question 7 of 30
7. Question
Find the HCF of 23,46,82723,46,827
Correct
Whenever we have to solve this sort of question, remember the formula.
HCF =HCF of Numerators LCM of Denominators HCF of Numerators LCM of Denominators
So answers will be option 1Incorrect
Whenever we have to solve this sort of question, remember the formula.
HCF =HCF of Numerators LCM of Denominators HCF of Numerators LCM of Denominators
So answers will be option 1 -
Question 8 of 30
8. Question
Find the LCM of 23,46,82723,46,827
Correct
Whenever we have to solve this sort of question, remember the formula.
LCM = \\begin{aligned} \\frac{HCF of Denominators}{LCM of Numerators} \\end{aligned}
So answers will be option 2,
Please also give attention to the difference in formula of HCF and LCMIncorrect
Whenever we have to solve this sort of question, remember the formula.
LCM = \\begin{aligned} \\frac{HCF of Denominators}{LCM of Numerators} \\end{aligned}
So answers will be option 2,
Please also give attention to the difference in formula of HCF and LCM -
Question 9 of 30
9. Question
Find the HCF of 2.1, 1.05 and 0.63
Correct
To solve this question quickly, first remove decimal by multiplying each term with 100,
Then terms become 210, 105, 63
Then HCF of above terms is 21,
So Answer is 0.21Incorrect
To solve this question quickly, first remove decimal by multiplying each term with 100,
Then terms become 210, 105, 63
Then HCF of above terms is 21,
So Answer is 0.21 -
Question 10 of 30
10. Question
If LCM of two number is 693, HCF of two numbers is 11 and one number is 99, then find other
Correct
For any this type of question, remember
Product of two numbers = Product of their HCF and LCMSo Other number =
693×1199693×1199
= 77
Incorrect
For any this type of question, remember
Product of two numbers = Product of their HCF and LCMSo Other number =
693×1199693×1199
= 77
-
Question 11 of 30
11. Question
LCM of two numbers is 7700 and HCF is 11. If one number is 308 then other number is
Correct
Incorrect
-
Question 12 of 30
12. Question
Which greatest possible length can be used to measure exactly 15 meter 75 cm, 11 meter 25 cm and 7 meter 65 cm
Correct
Convert first all terms into cm.
i.e. 1575 cm, 1125cm, 765cm.Now whenever we need to calculate this type of question, we need to find the HCF. HCF of above terms is 255.
Incorrect
Convert first all terms into cm.
i.e. 1575 cm, 1125cm, 765cm.Now whenever we need to calculate this type of question, we need to find the HCF. HCF of above terms is 255.
-
Question 13 of 30
13. Question
Find the greatest number which on dividing 1661 and 2045, leaves a reminder of 10 and 13 respectively
Correct
In this type of question, its obvious we need to calculate the HCF, trick is
HCF of (1661 - 10) and (2045 -13)
= HCF (1651, 2032) = 127Incorrect
In this type of question, its obvious we need to calculate the HCF, trick is
HCF of (1661 - 10) and (2045 -13)
= HCF (1651, 2032) = 127 -
Question 14 of 30
14. Question
Find the largest number which divides 62,132,237 to leave the same reminder
Correct
Trick is HCF of (237-132), (132-62), (237-62)
= HCF of (70,105,175) = 35Incorrect
Trick is HCF of (237-132), (132-62), (237-62)
= HCF of (70,105,175) = 35 -
Question 15 of 30
15. Question
Find the largest number of four digits which is exactly divisible by 27,18,12,15
Correct
LCM of 27-18-12-15 is 540.
After dividing 9999 by 540 we get 279 remainder.
So answer will be 9999-279 = 9720Incorrect
LCM of 27-18-12-15 is 540.
After dividing 9999 by 540 we get 279 remainder.
So answer will be 9999-279 = 9720 -
Question 16 of 30
16. Question
Six bells commence tolling together and toll at the intervals of 2,4,6,8,10,12 seconds resp. In 60 minutes how many times they will toll together.
Correct
LCM of 2-4-6-8-10-12 is 120 seconds, that is 2 minutes.
Now 60/2 = 30
Adding one bell at the starting it will 30+1 = 31Incorrect
LCM of 2-4-6-8-10-12 is 120 seconds, that is 2 minutes.
Now 60/2 = 30
Adding one bell at the starting it will 30+1 = 31 -
Question 17 of 30
17. Question
An electronic device makes a beep after every 60 sec. Another device makes a beep after every 62 sec. They beeped together at 10 a.m. The time when they will next make a beep together at the earliest, is
Correct
L.C.M. of 60 and 62 seconds is 1860 seconds
1860/60 = 31 minutesThey will beep together at 10:31 a.m.
Sometimes questions on red lights blinking comes in exam, which can be solved in the same way
Incorrect
L.C.M. of 60 and 62 seconds is 1860 seconds
1860/60 = 31 minutesThey will beep together at 10:31 a.m.
Sometimes questions on red lights blinking comes in exam, which can be solved in the same way
-
Question 18 of 30
18. Question
The greatest possible length which can be used to measure exactly the lengths 7 m, 3 m 85 cm, 12 m 95 cm is
Correct
So by now, you must be knowing this is a question of HCF, right.
H.C.F. of (700 cm, 385 cm, 1295 cm) = 35 cmIncorrect
So by now, you must be knowing this is a question of HCF, right.
H.C.F. of (700 cm, 385 cm, 1295 cm) = 35 cm -
Question 19 of 30
19. Question
The ratio of two numbers is 3 : 4 and their H.C.F. is 4. Their L.C.M. is
Correct
Let the numbers be 3x and 4x. Then, their H.C.F. = x. So, x = 4.
So, the numbers 12 and 16.
L.C.M. of 12 and 16 = 48Incorrect
Let the numbers be 3x and 4x. Then, their H.C.F. = x. So, x = 4.
So, the numbers 12 and 16.
L.C.M. of 12 and 16 = 48 -
Question 20 of 30
20. Question
The H.C.F. and L.C.M. of two numbers are 12 and 5040 respectively If one of the numbers is 144, find the other number
Correct
Solve this question by using below formula.
Product of 2 numbers = product of their HCF and LCM144 * x = 12 * 5040
x = (12*5040)/144 = 420
Incorrect
Solve this question by using below formula.
Product of 2 numbers = product of their HCF and LCM144 * x = 12 * 5040
x = (12*5040)/144 = 420
-
Question 21 of 30
21. Question
Find the greatest number that will divide 400, 435 and 541 leaving 9, 10 and 14 as remainders respectively
Correct
Answer will be HCF of (400-9, 435-10, 541-14)
HCF of (391, 425, 527) = 17
Incorrect
Answer will be HCF of (400-9, 435-10, 541-14)
HCF of (391, 425, 527) = 17
-
Question 22 of 30
22. Question
If the product of two numbers is 84942 and their H.C.F. is 33, find their L.C.M
Correct
HCF * LCM = 84942, because we know
Product of two numbers = Product of HCF and LCM
LCM = 84942/33 = 2574
Incorrect
HCF * LCM = 84942, because we know
Product of two numbers = Product of HCF and LCM
LCM = 84942/33 = 2574
-
Question 23 of 30
23. Question
Find the greatest number that will divide 43, 91 and 183 so as to leave the same remainder in each case. Correct
Required number = H.C.F. of (91 - 43), (183 - 91) and (183 - 43)
= H.C.F. of 48, 92 and 140 = 4.
Incorrect
Required number = H.C.F. of (91 - 43), (183 - 91) and (183 - 43)
= H.C.F. of 48, 92 and 140 = 4.
-
Question 24 of 30
24. Question
The H.C.F. of two numbers is 23 and the other two factors of their L.C.M. are 13 and 14. The larger of the two numbers is:
Correct
Clearly, the numbers are (23 x 13) and (23 x 14).
 Larger number = (23 x 14) = 322.Incorrect
Larger number = (23 x 14) = 322.Incorrect
Clearly, the numbers are (23 x 13) and (23 x 14).
 Larger number = (23 x 14) = 322.
Larger number = (23 x 14) = 322. -
Question 25 of 30
25. Question
Six bells commence tolling together and toll at intervals of 2, 4, 6, 8 10 and 12 seconds respectively. In 30 minutes, how many times do they toll together ? Correct
L.C.M. of 2, 4, 6, 8, 10, 12 is 120.
So, the bells will toll together after every 120 seconds(2 minutes).
In 30 minutes, they will toll together 30 + 1 = 16 times. 2 Incorrect
L.C.M. of 2, 4, 6, 8, 10, 12 is 120.
So, the bells will toll together after every 120 seconds(2 minutes).
In 30 minutes, they will toll together 30 + 1 = 16 times. 2 -
Question 26 of 30
26. Question
Let N be the greatest number that will divide 1305, 4665 and 6905, leaving the same remainder in each case. Then sum of the digits in N is: Correct
N = H.C.F. of (4665 - 1305), (6905 - 4665) and (6905 - 1305)
= H.C.F. of 3360, 2240 and 5600 = 1120.
Sum of digits in N = ( 1 + 1 + 2 + 0 ) = 4
Incorrect
N = H.C.F. of (4665 - 1305), (6905 - 4665) and (6905 - 1305)
= H.C.F. of 3360, 2240 and 5600 = 1120.
Sum of digits in N = ( 1 + 1 + 2 + 0 ) = 4
-
Question 27 of 30
27. Question
The greatest number of four digits which is divisible by 15, 25, 40 and 75 is:
Correct
Greatest number of 4-digits is 9999.
L.C.M. of 15, 25, 40 and 75 is 600.
On dividing 9999 by 600, the remainder is 399.
 Required number (9999 - 399) = 9600.Incorrect
Required number (9999 - 399) = 9600.Incorrect
Greatest number of 4-digits is 9999.
L.C.M. of 15, 25, 40 and 75 is 600.
On dividing 9999 by 600, the remainder is 399.
 Required number (9999 - 399) = 9600.
Required number (9999 - 399) = 9600. -
Question 28 of 30
28. Question
The product of two numbers is 4107. If the H.C.F. of these numbers is 37, then the greater number is:
Correct
Let the numbers be 37a and 37b.
Then, 37a x 37b = 4107
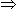 ab = 3.
ab = 3.Now, co-primes with product 3 are (1, 3).
So, the required numbers are (37 x 1, 37 x 3) i.e., (37, 111).
 Greater number = 111.Incorrect
Greater number = 111.Incorrect
Let the numbers be 37a and 37b.
Then, 37a x 37b = 4107
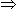 ab = 3.
ab = 3.Now, co-primes with product 3 are (1, 3).
So, the required numbers are (37 x 1, 37 x 3) i.e., (37, 111).
 Greater number = 111.
Greater number = 111. -
Question 29 of 30
29. Question
Three number are in the ratio of 3 : 4 : 5 and their L.C.M. is 2400. Their H.C.F. is
Correct
Let the numbers be 3x, 4x and 5x.
Then, their L.C.M. = 60x.
So, 60x = 2400 or x = 40.
 The numbers are (3 x 40), (4 x 40) and (5 x 40).
The numbers are (3 x 40), (4 x 40) and (5 x 40).Hence, required H.C.F. = 40.
Incorrect
Let the numbers be 3x, 4x and 5x.
Then, their L.C.M. = 60x.
So, 60x = 2400 or x = 40.
 The numbers are (3 x 40), (4 x 40) and (5 x 40).
The numbers are (3 x 40), (4 x 40) and (5 x 40).Hence, required H.C.F. = 40.
-
Question 30 of 30
30. Question
The G.C.D. of 1.08, 0.36 and 0.9 is:
Correct
Given numbers are 1.08, 0.36 and 0.90. H.C.F. of 108, 36 and 90 is 18,
 H.C.F. of given numbers = 0.18.Incorrect
H.C.F. of given numbers = 0.18.Incorrect
Given numbers are 1.08, 0.36 and 0.90. H.C.F. of 108, 36 and 90 is 18,
 H.C.F. of given numbers = 0.18.
H.C.F. of given numbers = 0.18.
